What is the Square Root Symbol?
Ever been puzzled by that unique-looking symbol used in mathematics to signify a square root? Wondered what secrets it holds or how it came to be?
It’s a symbol that crops up in many areas of math, from basic algebra to intricate equations in physics and engineering.
By the end of this post, you’ll have a greater appreciation for this cornerstone of mathematical notation and maybe, just maybe, you’ll see square roots in a new light.
So, are you ready to enlighten yourself?
Let’s get started!
Fast Facts
√
Square Root Symbol
Square Root Symbol (√) Overview
Here is a table that details the Square Root Symbol (√), including its Unicode information, description, and encodings:
| Attribute | Details |
|---|---|
| Symbol Name | Square Root |
| Unicode | U+221A |
| Image | √ |
| Brief Description | Represents the mathematical operation of finding the square root. |
| Unicode Version and Date | Unicode 1.1, June 1993 |
| Unicode Block Name | Mathematical Operators |
| Plane | Basic Multilingual Plane (BMP) |
| Script | Common |
| Category | Symbol, Math |
| Bidirectional Class | Other Neutrals (ON) |
| Combining Class | 0 |
| Character is Mirrored | No |
| HTML Entity | √ or √ or √ |
| CSS | \221A |
| UTF-8 Encoding | E2 88 9A |
| UTF-16 Encoding | 22 1A |
| UTF-32 Encoding | 00 00 22 1A |
Here’s What You Will Find
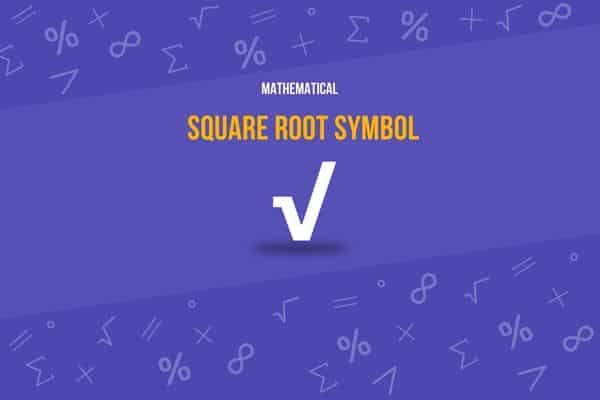
Square Root Symbol
The square root symbol, often represented as √, has a significant place in mathematics. It denotes the operation of finding the square root of a number, which is a value that, when multiplied by itself, gives the original number.
For example, the square root of 9 is 3 because 3×3 equals 9. This symbol originated from the Latin word ‘radix’, meaning root, and it’s therefore also called the radical symbol. Its widespread use in mathematics makes understanding it fundamental for many mathematical and scientific calculations. It’s found in various fields, from geometry to physics, engineering to computer science, proving its universal importance.
Other Names
Apart from being known as the square root symbol, this mathematical notation is often referred to as the radical sign or radical symbol. Derived from the Latin word ‘radix,’ which means ‘root,’ this term radical signifies its function to find the ‘root’ of a given number. When used to denote square roots specifically, it can be referred to as the “second root” symbol.
Additionally, in more advanced mathematics, when the symbol represents roots other than square roots, such as cube roots or fourth roots, it’s typically termed as the nth root symbol. The diversity in its naming reflects its wide-ranging utility in the world of numbers.
Square Root Symbol Meaning
The square root symbol, denoted as “√”, is a widely recognized mathematical symbol representing the operation of finding the square root of a number. The square root of a number x is another number y which, when multiplied by itself, equals x. In mathematical notation, this relationship is expressed as y2=xy2=x. The square root symbol itself is part of the broader family of radical symbols, used to denote roots of various degrees, though the square root is the most commonly encountered type.
The square root symbol, represented by ‘√’, signifies a mathematical operation of finding the square root of a given number or expression. A square root of a number is a value that, when multiplied by itself, yields the original number. For instance, the square root of 25 is 5 because 5 multiplied by 5 equals 25.
In a broader context, this symbol is not just limited to finding square roots but can also denote higher roots when a number (the index) is inserted into the ‘check’ of the symbol. For example, the cube root of 8 would be written as ‘∛8’, with the number ‘3’ indicating that we are looking for a number that, when multiplied by itself twice (i.e., cubed), equals 8.
The number or expression underneath the symbol is called the radicand. Sometimes, especially in more complex expressions, the square root symbol includes a horizontal line, known as the vinculum, over the radicand to clarify which numbers or variables are included in the operation.
Understanding the meaning of this symbol is crucial for studying mathematics. It allows us to solve equations, simplify expressions, and understand relationships between numbers at a deeper level.
Symbolism of the Square Root Symbol
The square root symbol, “√”, transcends its mathematical functionality, embodying deeper symbolic meanings in various contexts. Its primary association is with the concept of fundamental change or transformation, as it signifies the process of extracting a root, fundamentally altering the original number.
Representation of Balance and Harmony
In a more abstract sense, the square root symbol represents balance and harmony. This is evident in its application in geometry and algebra, where it often plays a crucial role in finding equilibrium points, such as the center of a circle or the solution to equations that describe balanced forces.
Symbol of Discovery and Insight
The square root operation can also symbolize the act of exploring beneath the surface, uncovering underlying truths. This is akin to solving a puzzle or revealing hidden knowledge. In educational contexts, it frequently marks significant moments of understanding or insight as students grasp the principles of radical operations.
Metaphor for Growth and Potential
Additionally, the square root symbol is a metaphor for growth and potential in various fields. In finance and economics, for example, it helps in calculating potential risks and returns, embodying the idea of exploring possibilities and outcomes.
The square root symbol thus holds a rich tapestry of meanings, from its concrete applications in solving mathematical problems to its metaphorical representation of deeper, philosophical concepts of balance, discovery, and growth.
Square Root Symbol Unicode
Unicode is a universal standard for the consistent encoding, representation, and handling of text expressed in most of the world’s writing systems. It’s designed to facilitate the use and display of characters from different languages and various symbols on digital platforms, essentially making it possible for us to share, view, and use text or symbols from around the globe without distortion or loss in translation.
The square root symbol’s Unicode is U+221A. The ‘U+’ denotes a Unicode character, and ‘221A’ is the hexadecimal number representing the character in the Unicode standard. When this code is used in a digital platform or programming language that supports Unicode, it will display the square root symbol (√), thereby enabling the clear and accurate representation of mathematical expressions involving square roots.
Square Root Symbol Uses
The square root symbol, “√”, serves as a cornerstone in various fields of mathematics and its applications. Understanding its uses provides insights into the broad impact of this mathematical symbol across different disciplines and everyday calculations.
Basic Arithmetic and Algebra
In primary mathematics, the square root symbol is used to solve quadratic equations where determining the square root of a number is necessary to find the values of unknown variables. For example, solving the equation ( x^2 = 16 ) involves finding the square root of 16, leading to ( x = ±4 ).
Advanced Mathematics
In more advanced mathematics, such as calculus and differential equations, the square root symbol appears in formulas and solutions where rates of change and other dynamic conditions are modeled. For instance, the standard deviation formula in statistics, a critical measure of variability or volatility, employs the square root to aggregate a dataset’s variance.
Engineering and Physics
Engineers and physicists often use the square root symbol when applying the Pythagorean theorem to determine distances or when calculating forces in mechanics under root laws, like the inverse square law that describes the intensity of light or gravity.
Finance
In finance, the square root is utilized in various models and formulas, including the assessment of the standard deviation of stock returns as a measure of volatility. For instance, the Black-Scholes model for pricing options uses the square root in its formulation to account for the volatility component over time.
Computer Science
Calculating square roots can be fundamental in algorithms and computational methods. It can help solve problems that require precise square root calculations, such as digital signal processing or graphics rendering.
Everyday Use
Beyond these specialized fields, the square root symbol finds utility in everyday contexts such as in construction, where determining the diagonal length of a square space might require the square root operation. It’s also common in educational settings, where students learn to manipulate and understand square roots as part of their curriculum in math classes.
These examples underscore the versatility and fundamental nature of the square root symbol in theoretical mathematics and practical applications across various scientific, engineering, and financial fields. The symbol itself facilitates the expression and solving of equations that form the backbone of many modern technologies and theories.
Square Root Symbol Examples
The square root symbol, “√”, is pivotal in many mathematical expressions and equations. Here are several practical examples that showcase the diverse applications of this symbol across different contexts:
Solving Quadratic Equations
One of the most common uses of the square root symbol is in solving quadratic equations. For instance, in the equation (x^2 = 49), the solution for (x) involves taking the square root of both sides to get (x = \pm7).
Calculating Diagonal of a Square
To find the diagonal length of a square with side length (s), you use the formula (d = s\sqrt{2}). This formula derives from the Pythagorean theorem and is widely used in architecture and design.
Standard Deviation in Statistics
In statistics, the standard deviation formula is ( \sigma = \sqrt{\frac{1}{N} \sum_{i=1}^N (x_i – \mu)^2} ), where (\sigma) is the standard deviation, (N) is the number of elements, (x_i) represents each element, and (\mu) is the mean of the elements. This usage highlights how the square root symbol helps quantify the spread or variability of a dataset.
RMS (Root Mean Square) Calculations
In engineering, particularly in electrical engineering, the root mean square (RMS) value of an alternating current (AC) is calculated using the square root symbol: ( RMS = \sqrt{\frac{1}{T} \int_0^T [f(t)]^2 dt} ). This value is critical for understanding the effective or average value of an AC current or voltage.
Geometric Mean
The geometric mean of a dataset is calculated using the square root symbol when the dataset contains two numbers, say (a) and (b). The geometric mean is (\sqrt{ab}), which is particularly useful in financial contexts where growth rates are compounded, such as in population studies or investment returns.
Distance Formula
In geometry, the distance between two points ((x_1, y_1)) and ((x_2, y_2)) in a coordinate plane can be determined using the square root symbol in the formula (d = \sqrt{(x_2 – x_1)^2 + (y_2 – y_1)^2}). This formula is an application of the Pythagorean theorem in a two-dimensional space.
These examples demonstrate the square root symbol’s critical role in various mathematical formulations and real-world applications, from simple arithmetic to complex statistical and engineering calculations. Each instance highlights the symbol’s utility in simplifying and solving equations across various disciplines.
Why is the Square Root Symbol Important?
The square root symbol is of paramount importance because it’s a fundamental tool in mathematics. It helps unlock solutions in an array of mathematical equations, from basic algebra to complex calculus. It allows us to express the ‘root’ concept clearly and concisely, thereby facilitating deeper understanding and exploration of mathematical relationships and patterns.
Beyond pure mathematics, the symbol plays a pivotal role in diverse fields such as physics, engineering, computer science, and statistics, underpinning critical calculations and analyses. In essence, the square root symbol provides an essential key to understanding and solving numerous problems across a wide variety of disciplines, making it a cornerstone of mathematical notation.
Square Root Symbol History
The square root symbol, “√”, has a fascinating historical development that traces back several centuries. Understanding its origins and evolution provides insight into how mathematical notation influences mathematical thinking and communication.
Early Beginnings
The concept of finding square roots can be traced back to the ancient civilizations of Babylon and Egypt, where they applied geometrical methods to construct square roots. However, the symbol that we recognize today has its origins in the European Renaissance.
The Symbol’s Evolution
The modern square root symbol evolved from the letter “r,” which stood for “radix” (meaning root in Latin). Its first recorded use in its approximate modern form was by the German mathematician Christoph Rudolff in his book “Coss” published in 1525. Rudolff’s symbol was not exactly the radical symbol we use today but was rather a stylized form of the letter “r”. This symbol was gradually refined over time.
Adoption and Standardization
The symbol gained more widespread use after being adopted by René Descartes in his “La Géométrie” in 1637, where he used a similar notation for roots. This helped to standardize the notation, although it continued to evolve. For a long time, the length of the horizontal line over the radicand (the number under the square root symbol) varied depending on the length of the numbers involved.
Refinement in the 17th and 18th Centuries
During the 17th and 18th centuries, mathematicians and typesetters experimented with various ways to depict the radical symbol, leading to the more uniform notation used today. This period was marked by an increased formalization of mathematical symbols and notations, driven by the proliferation of mathematical texts and the need for consistent communication of mathematical ideas across Europe.
Modern Usage
By the 19th century, the notation had largely standardized to the form we use today, with the “√” symbol followed by a vinculum (the horizontal bar) extending over the radicand. This form was widely adopted in mathematical education and is now universally recognized in mathematical and scientific disciplines.
The history of the square root symbol illustrates not just the evolution of a mathematical notation but also reflects broader changes in the field of mathematics, including the shift towards a more formal and standardized approach to mathematical expressions. It shows how symbols can evolve over time to meet the needs of clarity, precision, and ease of use in mathematical communication.
Square Root Symbol Origin
The square root symbol, “√”, widely recognized in mathematics for denoting the square root operation, has an interesting origin that dates back several centuries. The development of this symbol is intertwined with the evolution of mathematical notation and the needs of mathematicians to communicate complex operations clearly and succinctly.
Latin Roots
The symbol itself traces its origins to the Latin word “radix,” which means root. The square root symbol evolved from the abbreviation of “radix” to just its initial letter “r. Over time, this “r” was stylized to resemble the symbol we recognize today.
Christoph Rudolff’s Contribution
The modern square root symbol first appeared approximately in its current form in the book “Coss” by the German mathematician Christoph Rudolff, published in 1525. Rudolff’s use of the symbol was an innovation aimed at simplifying the notation for extracting roots, which was previously a cumbersome process written out in words or less standardized symbols. His symbol, a slightly sloped and elongated “r,” was designed for easier use in mathematical calculations and typesetting.
Evolution through Descartes
The symbol was further popularized and underwent standardization through its adoption by René Descartes, the French philosopher and mathematician, in his influential work “La Géométrie” in 1637. Descartes used a similar radical symbol, which helped to standardize its form and encourage its adoption across Europe. His influence in mathematics and the widespread distribution of his works played a crucial role in the symbol’s adoption.
Standardization in Mathematical Notation
As mathematical texts became more common and printing technology spread throughout Europe, the need for a clear and universally understandable set of symbols became apparent. The square root symbol, with its distinctive shape and clear implication, was an ideal candidate for this role. Over the centuries, its design was refined to enhance readability and simplicity, leading to today’s standardized form.
The Role of Typesetting
The evolution of typesetting technology also influenced the standardized appearance of the square root symbol. The need for a clear and concise way to represent the operation over a potentially long radicand (the number under the square root) led to the incorporation of the vinculum, or horizontal bar, over the radicand. This not only clarified the extent of the operation but also aligned with the emerging practices in mathematical notation for clarity and precision.
The origin of the square root symbol highlights the dynamic interplay between mathematical innovation, linguistic influences, and technological advancements in typesetting and printing. It stands as a testament to the ways in which mathematical notation evolves to meet the practical needs of its users, facilitating clearer communication and understanding across the mathematical community.
Evolution of the Square Root Symbol
The square root symbol has undergone an evolution since its inception in the 16th century. Christoff Rudolff, the German mathematician who first introduced the symbol, used it in a slightly different form than what we recognize today.
In Rudolff’s usage, the symbol was often more horizontally elongated and lacked the vinculum (the horizontal bar over the radicand). This original version, therefore, looked like a more elongated checkmark and was quite different from our current, compact ‘√’ symbol.
Did You Know?
The modern square root symbol evolved from the letter “r,” which stood for “radix” (meaning root in Latin).
As mathematical notation started to become more standardized over the centuries, the symbol evolved and became more uniform in appearance, leading to the square root symbol we are familiar with today.
The vinculum was added to clearly indicate which numbers or expressions were under the radical, enhancing clarity and preventing misinterpretation. While the changes may seem minor visually, they’ve had a significant impact on the symbol’s usability and universality in mathematical expression.
Square Root Symbol In Everyday Life
Beyond its fundamental mathematical purpose, the square root symbol has a philosophical significance that we can incorporate into our daily lives. The essence of this symbol is the idea of balance and symmetry, as it represents finding a number that, when multiplied by itself, produces the original number. This notion of balance can be a guiding principle in our daily routines.
Think about maintaining a healthy lifestyle, for example. Regular exercise (one part of the ‘equation’) combined with a balanced diet (the other part) together contribute to overall wellness (the ‘total’). The principle here is similar to the symmetry in a square root operation, where two identical factors combine to form a whole.
Or consider time management: splitting your time effectively between work, family, leisure, and personal growth mirrors the concept of finding that ‘square root’—the balance that when multiplied, creates the most fulfilling life.
Ultimately, the square root symbol can remind us that just as balance and symmetry are vital in equations, they are equally important in our lives, encouraging us to find harmony between our different roles and responsibilities.
Last Thoughts
The square root symbol, with its rich history, varied uses, and deep meaning, is more than just a mathematical notation—it’s an intriguing sign that has permeated many layers of our culture and daily life.
As we’ve seen, its principles can offer valuable insights into our routines and decision-making. From the 16th century to the present day, it has left a profound influence on multiple disciplines, not just within mathematics but also in the arts, pop culture, and personal philosophy.
Before You Go
Don’t stop your journey of discovery here—we encourage you to explore and appreciate the fascinating world of other symbols, each with its unique history, meaning, and significance in various fields of knowledge.
If you found this exploration into the history and uses of the square root symbol enlightening, why not share this knowledge with others?
Whether you’re a math enthusiast, a student, or just curious about the symbols we encounter in everyday life, passing along this post can spark interesting conversations and deepen your appreciation for the intricate world of mathematics. Share this post with friends, family, or colleagues, and help spread the fascination with this iconic mathematical symbol!













