What is the Division Symbol?
Are you curious about the ubiquitous “÷” division symbol we encounter in mathematics?
This post explores the rich history, various uses, and the origin of this essential mathematical symbol. From its earliest notations in ancient manuscripts to its current role in education and computing, we’ll take you through a detailed journey of how the division symbol became a fundamental part of mathematical language.
Let’s uncover the intriguing evolution of this iconic symbol!
Let’s get started!
Fast Facts
÷
Airplane Symbol
Division Symbol (÷) Overview
Here’s a detailed table that encapsulates a wide range of information about the Division Symbol (÷):
| Attribute | Description |
|---|---|
| Symbol Name | Division Sign |
| Unicode | U+00F7 |
| Image | ÷ |
| Brief Description | Used to represent the mathematical operation of division. |
| Unicode Version and Date | Introduced in Unicode 1.1.0 (June 1993) |
| Unicode Block Name | Latin-1 Supplement |
| Plane | Basic Multilingual Plane |
| Script | Common |
| Category | Symbol, Math |
| Bidirectional Class | European Separator (ES) |
| Combining Class | 0 (Not Reordered) |
| Character is Mirrored | No |
| HTML Entity | ÷ or ÷ |
| CSS | \00F7 |
| UTF-8 Encoding | 0xC3 0xB7 |
| UTF-16 Encoding | 0x00F7 |
| UTF-32 Encoding | 0x000000F7 |
| ASCII Code | Not applicable (outside the ASCII range) |
Here’s What You Will Find
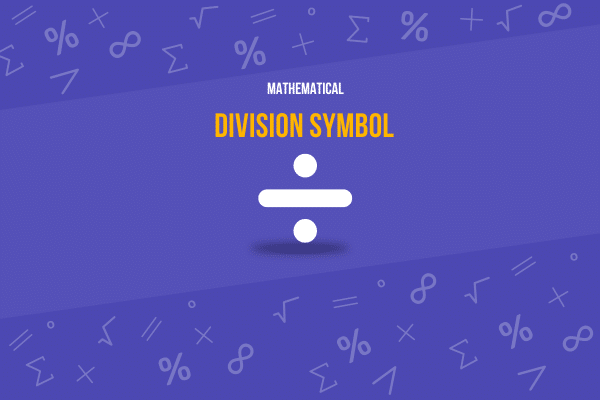
Division Symbol
The division symbol consists of a horizontal line with dots above and below, widely used to represent the operation of a division. In the statement “a ÷ b = c,” ‘a’ is the dividend, ‘b’ is the divisor, and ‘c’ is the quotient.
Despite its ubiquity in elementary arithmetic, it’s interesting that the division symbol is used less frequently in higher-level math and science, often replaced with a slash (/) or a horizontal line. Despite this, division is a vital tool, aiding our understanding of one of the most fundamental operations in mathematics.
Other Names
The division symbol is known by various names worldwide, each carrying a unique historical or mathematical context. Most commonly, it’s referred to as the obelus, a term originating from the Greek word ‘obelos,’ which means ‘a pointed stick’ or ‘a roasting spit.’ This name was originally used to refer to a printing mark (†) in ancient manuscripts, but later, it came to signify the division symbol in mathematics.
In addition, some educators and mathematicians call it the ‘division sign,’ aligning its name with other mathematical operations like the plus sign or minus sign. In a more informal context, it is often called the ‘divide symbol’ or ‘divide sign.’ Regardless of the name, its role in representing the mathematical operation of division remains constant across different languages and cultures.
Division Symbol Meaning
The division symbol, or obelus (÷), is a mathematical symbol primarily used to signify division operation. It features two dots, one placed above and the other below a horizontal line. The number preceding the symbol is known as the dividend—the quantity to be divided—and the number following it is called the divisor—the number by which the dividend is divided. The result of this operation is the quotient.
For example, in the expression “12 ÷ 3 = 4”, 12 is the dividend, 3 is the divisor, and 4 is the quotient. Beyond elementary arithmetic, the symbol can represent the concept of ‘per’ in certain contexts, like “miles per hour” in speed calculations. It can also be used to express ratios or fractions. Despite its ubiquity in everyday mathematics, it’s worth noting that in higher-level mathematics, the division symbol is often replaced by a forward slash (/) or a fraction bar for brevity and convenience. While its usage varies somewhat across different fields and levels of mathematics, the fundamental meaning of the division symbol as a marker of the division operation remains constant.
Symbolism of the Division Symbol
The division symbol (÷), also known as the obelus, carries rich layers of symbolism beyond its primary mathematical function. Traditionally used to indicate the operation of division, its form—a line flanked by two dots—suggests a sense of separation and allocation, themes that resonate deeply in various cultural and philosophical contexts.
Mathematical and Educational Symbolism
In mathematics, the division symbol represents the fundamental concept of dividing a whole into parts, a crucial operation in arithmetic, algebra, and beyond. It symbolizes the distribution of resources or elements equally and is often used to teach concepts of fairness and sharing. This educational use imbues the symbol with notions of equality and justice, as it helps students understand how to divide things evenly among a number of people or factors.
Philosophical and Cultural Interpretations
Philosophically, the division symbol represents the dichotomy between unity and duality. It visually and functionally splits a single entity into multiple parts, mirroring life’s many dualities—such as light and dark, good and evil, or past and future. This aspect makes the symbol a tool for discussing balance and the inherent divisions within nature and human experience.
Visual and Artistic Impressions
Visually, the division symbol’s simple yet powerful design evokes a pathway or a bridge between two points or concepts linked by the central line. In art and design, it can be used metaphorically to explore themes of connection and separation, making it a versatile element in visual storytelling.
Overall, the division symbol serves not only as a tool for calculation but also as a profound emblem of division and connection, balance, and fairness. Its symbolism extends into realms of philosophy, culture, and art, where it continues to inspire deeper thought about the nature of dividing and sharing both tangible and abstract entities.
Division Symbol Unicode
Unicode is a universal standard that provides a unique number for every character or symbol, regardless of the platform, program, or language used. This international encoding system is designed to encompass all characters from all writing systems worldwide, thus facilitating digital communication and ensuring compatibility across different systems.
The Unicode for the division symbol (÷) is U+00F7. The ‘U+’ signifies that what follows is a Unicode value, and the ’00F7′ is the hexadecimal representation of the number corresponding to the division symbol. So, wherever you are in the digital world, if you want to include a division symbol, simply using the Unicode value U+00F7 will ensure it is recognized and displayed correctly
Division Symbol Uses
The division symbol (÷) is critical in various mathematical contexts and beyond.
The division symbol, represented as “÷”, is integral to mathematics. It serves as a clear and concise notation for division operations. Its uses extend across various fields and applications, emphasizing its versatility and importance.
Basic Arithmetic
The primary use of the division symbol is in basic arithmetic, where it indicates the division of numbers. For example, in the equation “12 ÷ 3 = 4”, the division symbol tells us to divide 12 by 3, resulting in 4. This operation is fundamental in teaching mathematics at the elementary level, helping students understand the concept of division as sharing or grouping.
Algebra
In algebra, the division symbol simplifies expressions and solves equations involving variables. For instance, the equation “x ÷ 5 = 10” can be solved to find the value of x by multiplying both sides by 5. This application makes the division symbol crucial in manipulating and simplifying algebraic expressions.
Fractions
The division symbol is closely related to fractions. A fraction is an expression of division. The fraction “a/b” is another way of writing “a ÷ b.” This shows the division of ‘a’ by ‘b’. Understanding this relationship is key in converting between fractions and division expressions, a common task in basic arithmetic and more advanced mathematics.
Calculators and Computing
The division symbol is used to perform division operations on calculators and in various software applications. In programming and spreadsheet software, such as Python or Excel, the division symbol (typically “/”) is employed to calculate quotients or create formulas involving division. This utility makes the division symbol indispensable in fields that require precise calculations, such as engineering, finance, and data analysis.
Mathematical Proofs and Theorems
In more advanced branches of mathematics, such as calculus and number theory, the division symbol is used within proofs and theorems to denote division operations necessary for establishing certain mathematical truths. Its presence in such contexts underscores its fundamental role in the broader mathematical discipline.
Educational Tools and Resources
In educational contexts, the division symbol is prominently featured in textbooks, workbooks, and digital learning tools to teach and reinforce division. Its consistent use helps students from early education to higher learning grasp the concept of division clearly and effectively.
The division symbol, therefore, is not just a method of indicating a mathematical operation but a bridge connecting various levels and branches of mathematics. Its simplicity in form belies its extensive utility and fundamental nature in the world of numbers and beyond.
Besides this basic application, the division symbol is also employed in teaching fundamental math concepts to young learners, as it is more visually distinct and easier to grasp than the fraction bar or the forward slash.
Division Symbol Examples
To illustrate the practical application and importance of the division symbol in various mathematical contexts, here are several examples that demonstrate its use. These examples span basic arithmetic, algebra, and real-world applications, providing a comprehensive view of how the division symbol facilitates mathematical operations.
Basic Arithmetic Examples
- Simple Division:
- Example: 24 ÷ 6 = 4
- Explanation: This equation uses the division symbol to indicate that 24 is being divided by 6, resulting in 4.
- Complex Divisor:
- Example: 100 ÷ 25 = 4
- Explanation: Here, the division symbol shows a division operation where 100 is divided by 25, yielding a quotient of 4.
- Decimal Results:
- Example: 7 ÷ 2 = 3.5
- Explanation: The division of 7 by 2 does not result in a whole number, demonstrating that the division symbol can also lead to decimal results, not just integers.
Algebraic Examples
- Solving for Variables:
- Example: ( x ÷ 5 = 10 ) (solve for x)
- Explanation: This equation can be solved by multiplying both sides by 5, demonstrating the use of the division symbol in isolating and solving for variables in algebraic equations.
- Expressions with Variables:
- Example: ( \frac{y + 7}{3} = y ÷ 3 + 7 ÷ 3 )
- Explanation: The division symbol is used to simplify expressions involving variables, showing its utility in breaking down more complex algebraic expressions into simpler terms.
Real-World Application Examples
- Budgeting:
- Example: If your monthly income is $3000 and you spend $500 on groceries, then ( \frac{3000}{500} = 6 ) indicates you can allocate your income into 6 equal parts of $500 each.
- Explanation: The division symbol helps in financial planning by determining how many times a certain amount can fit into another, facilitating better budget management.
- Baking and Cooking:
- Example: A recipe requires 250 grams of flour, and the chef uses a 50-gram measuring cup. The calculation ( 250 ÷ 50 = 5 ) tells the chef they need to use the cup five times.
- Explanation: This practical application of the division symbol in everyday life highlights its importance in tasks like cooking and baking, where precise measurements are crucial.
- Time Management:
- Example: If a lecture lasts 120 minutes and consists of 4 sections, then ( 120 ÷ 4 = 30 ) minutes per section.
- Explanation: The division symbol allocates time evenly across different segments of an event or task, ensuring efficient time management.
These examples demonstrate the utility of the division symbol in educational and everyday contexts. It serves as a tool for teaching and learning mathematics and as an essential element in daily decision-making and problem-solving activities.
Why is the Division Symbol Important?
The division symbol (÷) holds significant importance in mathematics and beyond. Its foremost role is to represent the operation of division—one of the four basic operations of arithmetic—thereby providing a universally recognized way to denote this fundamental process. Understanding division, and thus the division symbol, is critical in various areas of life, from sharing items fairly to calculating rates, averages, and probabilities.
The division symbol also plays an instrumental role in education, helping students comprehend the concept of division clearly and visually. Furthermore, the symbol is essential for conveying mathematical ideas accurately and consistently across different platforms and languages. Without it, communication of mathematical concepts would become far more complicated, especially in a digital context. Hence, the division symbol is a crucial tool in mathematics and an integral part of our everyday numeracy.
Division Symbol History
The history of the division symbol is a fascinating journey through time and mathematics, revealing how a simple mark evolved into a universal mathematical operator. Understanding the origins and historical development of the division symbol “÷” provides insights into the evolution of mathematical notation and its impact on the world.
Ancient Beginnings
The concept of division is as old as mathematics, with ancient civilizations such as the Egyptians and Babylonians using their unique methods and symbols to perform division. However, these early forms did not include the modern division symbol. They often used repeated subtraction or other labor-intensive methods documented on papyrus or clay tablets.
Introduction of the Obelus
The symbol we recognize today as the division symbol was first used by the Swiss mathematician Johann Rahn in his book “Teutsche Algebra,” published in 1659. The symbol, the obelus (÷), originally had a slightly different and broader use. In some earlier texts, it was used to signify subtraction and other mathematical operations.
Adoption in Mathematics
The adoption of the obelus as a division symbol is largely attributed to the influence of British mathematician Thomas Harriot. His posthumous publication, “Artis Analyticae Praxis ad Aequationes Algebraicas Resolvendas” (The Analytical Arts Applied to Solving Algebraic Equations), featured the obelus. This helped standardize its use in the division, particularly in English-speaking countries, during the 17th century.
Evolution and Standardization
Throughout the 18th and 19th centuries, the division symbol appeared more frequently in mathematical texts across Europe and America. As mathematics education became formalized, the need for a clear and universally recognizable set of symbols became apparent. The division symbol we use today became more standardized during this period, helping to simplify written mathematical expressions significantly.
The Symbol in Contemporary Times
Today, the division symbol is universally recognized and used in educational materials worldwide. It appears not only in traditional textbooks but also in digital formats on calculators and computer software. Despite introducing the slash (“/”) as an alternative notation for division in inline text and computing, the traditional obelus remains popular in educational contexts.
Symbolic Implications
The division symbol’s history is a technical evolution and reflects the growing need for efficient communication in mathematics. Its development from a mark used sporadically in manuscripts to a standard operator in textbooks and digital devices underscores the dynamic nature of mathematical notation.
In conclusion, the division symbol’s history is a testament to the adaptability and enduring nature of mathematical symbols. It highlights how a simple notation can evolve to meet the needs of generations of mathematicians and educators, becoming an indispensable part of the mathematical lexicon.
Division Symbol Origin
The division symbol, commonly known as the obelus (÷), has an origin story that stretches back to the annals of history, blending with developments in mathematics and typography. The evolution of this symbol is intertwined with the progression of mathematical notation, reflecting changes in how mathematicians and educators have approached the concept of division over the centuries.
Early Notations and the Role of Manuscripts
Originally, the concept of division was represented in various ways, depending on the culture and the era. Ancient mathematicians like the Egyptians and Babylonians used specific methods for division, often involving complex algorithms depicted in hieroglyphs or cuneiform inscriptions. These early notations were far from the simplicity of the modern division symbol and often required extensive explanations.
Johann Rahn and the Introduction of the Obelus
The obelus, as we know it, was first introduced by Johann Rahn in 1659 in his book “Teutsche Algebra.” However, the symbol itself predates Rahn. It was originally used by the Greek scholar and editor Zenodotus in the 3rd century BCE. In ancient texts, the obelus denoted a questionable or spurious passage in manuscripts, not division. It was a critical mark, resembling a dagger, which later evolved in appearance and application.
Adoption in Mathematical Texts
The transition of the obelus from a critical mark to a mathematical symbol is attributed primarily to its adoption by mathematicians in the 17th century. Thomas Harriot, an English mathematician, used it in his algebraic works to indicate subtraction and division. The symbol’s simplicity and clarity made it increasingly popular among mathematicians for denoting division, facilitating its spread across mathematical texts in Europe.
The Symbol’s Evolution
Over time, the obelus underwent slight modifications in style and usage. As mathematical printing developed, the symbol became more standardized, shifting from various dashes or slashes to the familiar line with dots above and below. This format was easier to print and more visually distinct, aiding in its adoption in educational materials.
Typographical Developments
The consolidation of the obelus as the division symbol in mathematics was also influenced by typographical advances during the 18th and 19th centuries. The need for a consistent and universally understandable mathematical notation drove the inclusion of the obelus in typographic sets used in mathematical publishing. This ensured that the symbol was readily available to printers and publishers, embedding it further into the fabric of mathematical notation.
Modern Adoption
In contemporary times, the division symbol remains a staple in mathematical notation, used in textbooks, calculators, and digital interfaces worldwide. Its design is immediately recognizable and serves as a fundamental tool in teaching basic and advanced mathematics.
Tracing the origin of the division symbol reveals a fascinating blend of historical, cultural, and technological influences that have shaped its development. From ancient critical marks to a cornerstone of modern mathematics, the division symbol’s journey is a testament to the evolving nature of mathematical expression and communication.
Evolution of the Division Symbol
Before the obelus became a mathematical symbol, it underwent several large transformations. It was originally only a plain line (−) before dots were added to it (⨪ and ÷). When Rahn adopted the symbol for use in mathematics, the version with two dots was chosen over the single and no-dot versions, making the two-dot version the most common version of the obelus today.
Division Symbol In Everyday Life
Reflecting on the division symbol and its underlying principles provides valuable insights to incorporate into our daily routines. Beyond its mathematical function, the division symbol embodies the idea of partitioning, distributing, or sharing equally—a concept with numerous real-world applications.
For instance, when planning our time, we could think of the total daily hours as the dividend and the number of tasks as the divisor. This approach allows us to ‘divide’ our time efficiently among our responsibilities, mirroring the function of the division operation in arithmetic.
Similarly, when considering shared expenses among a group of friends or family members, we can employ the concept of division to ensure everyone contributes an equal share. This principle can also apply to dividing resources or tasks within a team at work, promoting fairness and balance.
On a broader level, the division symbol’s principle can remind us of the importance of equal distribution and balance in various aspects of our lives, from managing resources to sharing responsibilities. As such, the division symbol and its ideas can be practical tools for organizing and understanding our everyday activities.
Last Thoughts
The division symbol is far more than just a mathematical notation. It is a vital part of our everyday numeracy, a symbol rich with history, and a concept with broader cultural influences and applications. It’s fascinating to think about how this simple symbol has evolved and plays an essential role in our lives. Inspired by the journey of the division symbol? Why not explore the fascinating world of symbols and signs, each with unique stories and meanings?
Before You Go
We hope you found this exploration of the division symbol enlightening and interesting! If you enjoyed reading about the fascinating journey of this mathematical symbol, please consider sharing this post with friends, family, or colleagues.
Spreading knowledge is a wonderful way to spark curiosity and inspire a deeper appreciation for the rich history behind the symbols we use every day.
Share today and start a conversation about the intriguing world of mathematical symbols!





















