What is the Cube Root Symbol?
Are you ready to unlock the secrets of the cube root symbol?
Today, we will explore the mystical symbol representing the cube root and reveal its hidden meaning.
From its origins to its practical applications, we’ll take you on a journey of discovery.
So join us as we unravel the mysteries of the cube root symbol.
Let’s get started!
Fast Facts
∛
Cube Root Symbol
Cube Root Symbol (∛) Overview
Here is a table that includes detailed information about the Cube Root Symbol (∛):
| Attribute | Detail |
|---|---|
| Symbol Name | Cube Root |
| Unicode | U+221B |
| Image | ∛ |
| Brief Description | Represents the cube root operation in mathematics, typically used to denote the cube root of a number. |
| Unicode Version and Date | Unicode 1.1, released in June 1993 |
| Unicode Block Name | Mathematical Operators |
| Plane | Basic Multilingual Plane (BMP) |
| Script | Common |
| Category | Symbol, Math |
| Bidirectional Class | Other Neutral (ON) |
| Combining Class | 0 (Not Reordered) |
| Character is Mirrored | No |
| HTML Entity | √ or ∛ or ∛ |
| CSS | \221B |
| UTF-8 Encoding | E2 88 9B |
| UTF-16 Encoding | 22 1B |
| UTF-32 Encoding | 00 00 22 1B |
| ASCII Code | Not applicable (outside ASCII range) |
Here’s What You Will Find
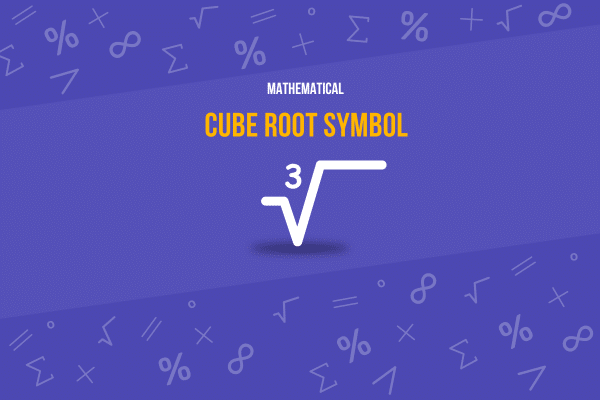
Cube Root Symbol
The cube root symbol (∛) is a mathematical notation that represents finding the number that equals a given value when multiplied by itself three times. It is a variation of the square root or radical symbol (√). The cube root symbol is a radical symbol with a small three on its left side, indicating the third root. It allows us to work with numbers and expressions involving cube roots concisely and efficiently.
Understanding the cube root symbol is a valuable tool for solving equations, analyzing geometric shapes, and exploring real-world problems.
Other Names
A few other mathematical names also know the cube root symbol (∛). One common alternative name for this symbol is the “radical symbol for cube root.” Since the cube root operation involves finding the principal root of a number, it is sometimes referred to as the “principal cube root symbol.”
In some contexts, it may be called the “cubic root symbol” or the “root symbol for cube.” Regardless of the name, this symbol remains a fundamental tool for working with cube roots and plays a significant role in various mathematical calculations and problem-solving scenarios.
Cube Root Symbol Meaning
The Cube Root Symbol (∛) is a mathematical symbol used to denote the cube root of a number. This symbol is expressed as ∛x, where “x” represents the number or expression of the cube root being calculated. In mathematical terms, finding the cube root of a number is the inverse operation of cubing a number.
For instance, if ∛27 is calculated, the result is 3 because 3³ (3 x 3 x 3) equals 27.
The Cube Root Symbol is part of the Unicode character set and is included under the Mathematical Operators block, which comprises various symbols used widely across mathematical expressions.
This symbol helps convey complex mathematical concepts succinctly, especially in algebra, geometry, and higher-level mathematics, where expressions involving roots are common. Its presence in educational texts, scientific documentation, and digital content enhances clarity and understanding, allowing mathematicians and students to communicate mathematical ideas more effectively.
Cube Root Symbol Unicode
Unicode is a universal character encoding standard that assigns a unique numeric code to every character, symbol, and script used in various writing systems worldwide. The Unicode for the cube root symbol (∛) is U+221B.
This code point is specifically reserved for the cube root symbol within the Unicode system. It allows computers and software applications to recognize and correctly display the cube root symbol, regardless of the operating system or font used. The Unicode standard has been instrumental in promoting internationalization and facilitating the exchange of information across different languages and cultures, making it an essential foundation for modern digital communication.
Cube Root Symbol Uses
The Cube Root Symbol (∛) is versatile in mathematics and its applications, extending its utility beyond theoretical calculations. Here are some of the primary uses of the cube root symbol:
- Solving Algebraic Equations: In algebra, the cube root symbol is frequently used to solve cubic equations where one needs to find a value of x such that (x^3 = a). For instance, in the equation (x^3 = 27), using the cube root, we find (x = \sqrt[3]{27} = 3).
- Real-World Measurements: The cube root is essential in calculations involving volume, where one dimension is sought from the volume of a cube-like structure. For example, to find the edge length of a cubic tank holding 1,000 cubic meters, one would calculate (\sqrt[3]{1000}) meters.
- Engineering: In engineering, cube roots derive formulas related to physical properties that depend on volume, such as density and pressure in thermodynamics.
- Geology and Earth Science: Cube roots are applied to estimate the strength of earthquakes based on the energy released and to model the formation of geological structures.
- Economics and Finance: In financial modeling and economics, cube roots can calculate investments’ annual growth rates or populations compounded for multiple periods.
- Statistics and Data Analysis: Cube roots are used in data transformations, helping to stabilize variance and make the data more suitable for analysis in cases involving cubic relationships.
- Computer Graphics: Cube root calculations are part of algorithms for generating 3D effects and animations where proportions and dimensions must be accurately rendered based on volume calculations.
- Education and Learning: The cube root symbol is a fundamental component of educational curricula in mathematics, helping students understand dimensions and the concept of inverse operations in algebra.
Each of these applications shows how the cube root symbol is a critical tool across various disciplines. It facilitates complex calculations and contributes to diverse scientific and practical fields.
The cube root symbol (∛) has applications in various fields, from mathematics to physics, engineering, and everyday life. In mathematics, the cube root symbol expresses the principal cube root of a number, allowing for a concise representation of complex calculations involving cubic roots. It is employed in solving equations, simplifying expressions, and manipulating algebraic equations involving cubic terms.
In physics, the cube root symbol is utilized in calculations related to volumetric quantities, such as finding the edge length of a cube with a given volume. Engineers often rely on the cube root symbol to solve problems related to scaling, such as determining the dimensions of objects or adjusting measurements in engineering designs.
Cube Root Symbol Examples
The Cube Root Symbol (∛) is a mathematical tool for solving problems and simplifying complex expressions and enhancing understanding in various contexts. Here are some practical examples that illustrate how the cube root symbol is applied:
Basic Mathematical Calculation
- Example 1: Calculate ∛64. The cube root of 64 is 4, because 4^3 = 4 × 4 × 4 = 64.
- Example 2: Determine ∛125. The answer is 5, since 5^3 = 5 × 5 × 5 = 125.
Scientific Applications
- Physics: If the volume of a cube is known to be 729 cubic meters, finding the length of a side of the cube involves calculating ∛729, which equals 9 meters.
- Chemistry: Knowing the volume allows chemists to work backward using the cube root to find linear dimensions necessary for further calculations when determining the concentration of substances in a cubic container.
Real World Scenarios
- Construction and Architecture: When planning the foundation for a large water tank that must hold 1,000 cubic feet of water, engineers calculate the cube root of 1,000 (∛1000 ≈ 10 feet) to determine whether each side’s length is perfectly cubic.
- Agriculture: Calculating the root volume necessary for the healthy growth of a tree might involve taking the cube root of the total soil volume available to the roots.
Educational Context
- Learning Exercises: Mathematics educators often use cube roots in problems to teach students about inverse operations and the relationships between exponents and roots. For example, asking students to find a number whose cube equals a given number (like solving ∛343) helps reinforce the concept of cubes and cube roots.
Advanced Mathematics
- Calculus and Analysis: In calculus, the cube root function is used to solve differential equations where variables are raised to the third power, such as finding the curve of a graph whose rate of increase follows a cubic proportionality.
Technology and Computing
- Algorithm Design: In computer science, algorithms that involve three-dimensional space calculations, such as those used in 3D modeling and virtual reality, often incorporate cube root computations to adjust dimensions and scale objects accurately.
These examples highlight the cube root symbol’s diverse applications across different fields, demonstrating its fundamental role in basic and advanced mathematical calculations and its practical importance in various scientific and real-life scenarios.
Why is the Cube Root Symbol Important?
The cube root symbol (∛) is significant in mathematics and beyond. It provides a concise and standardized notation for finding the principal cube root of a number. Mathematical expressions and equations involving cubic roots can be communicated and understood efficiently using the cube root symbol.
This symbol is invaluable in various mathematical disciplines, including algebra, calculus, and geometry. It enables mathematicians, scientists, and engineers to solve complex problems, simplify equations, and analyze cubic relationships. Furthermore, the cube root symbol finds practical applications in fields like physics, engineering, and data analysis, where understanding and manipulating cubic quantities are crucial.
Cube Root Symbol History
The history of the cube root symbol (∛) can be traced back to the development of mathematical notation and symbolism over centuries.
The history of the cube root symbol (∛) is intertwined with the development of mathematical notation over centuries. While the concept of cube roots can be traced back to ancient civilizations, the specific symbol we use today has evolved significantly over time. Here’s an exploration of how the cube root symbol came into existence and developed:
- Ancient Origins: The concept of cube roots dates back to ancient times, with early civilizations such as the Babylonians and Egyptians solving cubic equations for practical applications like land measurement and construction. These cultures did not have a specific symbol for cube roots but understood the mathematical principles behind cubic calculations.
- Classical and Medieval Periods: Greek mathematicians like Hero of Alexandria worked on methods to calculate cube roots in geometric problems. During the medieval period, Islamic scholars further developed algebra, including methods for solving cubic equations. The work of Al-Khwarizmi, a prominent 9th-century mathematician, includes algorithms for extracting cube roots, though still without a dedicated symbol.
- Renaissance Developments: The symbol for square roots (√) was introduced in the 16th century by Christoph Rudolff, but the cube root symbol took longer to appear in mathematical texts. It wasn’t until the 17th century that mathematicians began using variations of the radical symbol to indicate cube roots, often extending the “check” mark of the square root symbol with an additional stroke or using a small three to denote the concept (as in ∛x or ^3√x).
- Formal Adoption and Standardization: The notation ∛x, resembling a modified square root symbol, became more commonly used in the 18th and 19th centuries as mathematics formalized symbolic notation. It allowed a clear, concise representation of the operation, facilitating easier manipulation of expressions involving cube roots in algebra and calculus.
- Modern Usage: In the 20th century, with modern printing and typesetting technologies, the cube root symbol was standardized across mathematical textbooks and academic papers. The introduction of Unicode in the 1990s further solidified its representation in digital formats, ensuring that the symbol could be consistently used in electronic documents and computational software.
The history of the cube root symbol reflects broader trends in the evolution of mathematical notation. It showcases how symbols are tools for calculation and products of cultural and technological advancements that make mathematical ideas more accessible and universally understood. A
s mathematical concepts have become more standardized and globally recognized, symbols like the cube root have become indispensable in conveying complex mathematical ideas clearly and efficiently.
Cube Root Symbol Origin
The origin of the cube root symbol (∛) is a fascinating aspect of the history of mathematical notation. It reflects the evolution of mathematical practices and the increasing need for efficiency in symbolic representation. Understanding its origin provides insight into how mathematicians have historically tackled the challenge of expressing complex operations clearly and succinctly.
- Early Computational Methods: Before establishing a specific symbol for cube roots, ancient mathematicians from various cultures, including the Babylonians, Greeks, and Indians, had methods for finding cube roots. These methods were primarily verbal or written in lengthy descriptive forms without any standardized symbols.
- Introduction of Radical Notation: The concept of using a radical sign for roots is generally credited to the German mathematician Christoph Rudolff, who introduced the square root symbol (√) in 1525 in his book “Coss.” However, the cube root and higher roots did not have a standard notation and were often written out as textual descriptions.
- Evolution Towards Symbolism: As algebra developed during the Renaissance, mathematicians began experimenting with extending the radical notation to cube roots. The challenge was to differentiate the cube root from the square root in written form. This led to the adaptation of the square root symbol by extending its “leg” or adding a numeral above or before the radical to indicate the root’s degree. The notation for cube roots as seen today (∛), which resembles a modified square root symbol with an extended checkmark, began to appear more consistently in mathematical texts around the 17th century.
- Standardization in Mathematical Texts: By the 18th century, mathematical notation became more formalized, the cube root symbol was standardized in the form we recognize today. This was part of a broader movement towards a concise and universally understandable mathematical language, crucial for disseminating ideas as mathematical knowledge became more complex and widespread.
- Adoption in Modern Mathematics: This notation solidified in the 20th century as typesetting technologies improved, allowing for more consistent use of specialized symbols in printed materials. The formal inclusion of the cube root symbol in Unicode in the 1990s further cemented its place in digital and printed mathematics, making it a standard part of the global mathematical lexicon.
The origin of the cube root symbol reflects a blend of cultural innovation and the practical necessities of mathematical communication. It highlights how mathematical notation is not static but evolves in response to the needs of the community of mathematicians and educators, aiming to make the sharing and learning of mathematical concepts as efficient and universal as possible.
Evolution of the Cube Root Symbol
The cube root symbol (∛) has undergone changes and refinements in its representation over time. Its evolution can be observed through the development of mathematical notation and various mathematicians’ contributions. Initially, cube roots existed in ancient mathematics but without a standardized symbol.
These early symbols varied in appearance and often lacked the familiar index-like form we recognize today. Over time, through the efforts of mathematicians and the establishment of mathematical standards, the cube root symbol (∛) evolved to its current form—a radical symbol with a small three on its left side.
Cube Root Symbol In Everyday Life
The cube root symbol (∛) prompts personal reflections on the beauty and power of mathematics in our daily lives. It reminds us of the elegance and precision found in nature and scientific phenomena. Incorporating the principles the cube root symbol embodies into our daily routines can enhance our problem-solving and critical-thinking skills.
It encourages us to embrace complexity and seek solutions beyond superficial understanding. By incorporating these principles into our routines, we foster a mindset that values exploration, embraces complexity, and nurtures a lifelong love for learning and discovery.
Last Thoughts
The cube root symbol (∛) is a testament to the evolution of mathematical notation and its profound impact on the communication of complex mathematical concepts. From its ancient computational roots to its formalization in modern mathematics, this symbol exemplifies how mathematical symbols transcend mere placeholders to become powerful tools for conveying ideas succinctly and effectively.
The history and applications of the cube root symbol showcase not only the intellectual endeavors of mathematicians across centuries but also highlight the importance of clarity and efficiency in mathematical expression. As we continue to explore and teach mathematics, symbols like the cube root play a crucial role in bridging understanding across diverse fields and cultures, making mathematics a universal language.
Before You Go
If you find yourself captivated by the world of mathematical symbolism, explore the fascinating array of other symbols that await your discovery. We hope you found our exploration of the cube root symbol (∛) enlightening and engaging! If you enjoyed this journey through its history, applications, and significance, why not share this knowledge with others?
Passing on this post can spark curiosity and appreciation for the beauty of mathematical symbols among your friends, family, or colleagues. Whether they’re math enthusiasts, students, or simply lovers of learning, this post has something to offer everyone. Share today and help spread the fascination with the universal language of mathematics!





















