What is the Angle Symbol?
Have you ever pondered those seemingly simple yet surprisingly significant little angles in geometry? Let’s look into the incredible world of angles and their versatile symbol. This essential tool is fundamental in creating and understanding our three-dimensional world, from classroom chalkboards to advanced architectural blueprints.
As we progress, you’ll find that the more you comprehend this geometric marvel, the more your appreciation for its complexity and simplicity will grow.
So, why wait? Forge ahead and broaden your mathematical horizons with us today.
Let’s get started!
Fast Facts
∠
Angle Symbol
Angle Symbol (∠) Overview
Here’s a comprehensive table detailing the key attributes of the Angle Symbol (∠):
| Attribute | Details |
|---|---|
| Symbol Name | Angle Symbol |
| Unicode | U+2220 |
| Image | ∠ |
| Brief Description | Used in geometry to denote an angle. |
| Unicode Version and Date | Unicode 1.1, released in June 1993 |
| Unicode Block Name | Mathematical Operators |
| Plane | Basic Multilingual Plane (BMP) |
| Script | Common |
| Category | Symbol, Math |
| Bidirectional Class | Other Neutral (ON) |
| Combining Class | 0 |
| Character is Mirrored | No |
| HTML Entity | ∠ or ∠ |
| CSS | \2220 |
| UTF-8 Encoding | E2 88 A0 |
| UTF-16 Encoding | 2220 |
| UTF-32 Encoding | 00002220 |
Here’s What You Will Find
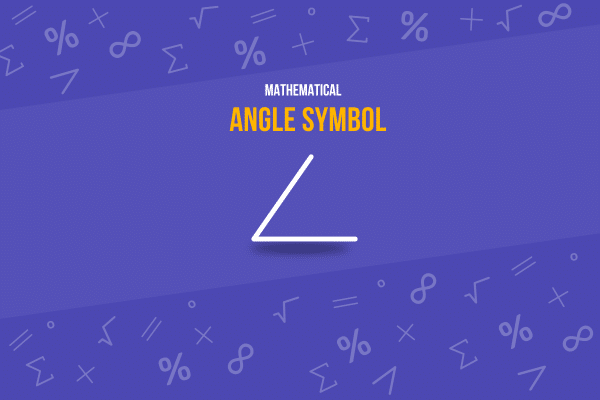
Angle Symbol
The angle symbol, often denoted as ∠ in mathematics, is a fundamental concept used to express the measure of an angle, the figure formed by two rays, called the sides of the angle, sharing a common endpoint, known as the angle’s vertex.
When referenced, the symbol is usually followed by three letters representing points that form the angle, with the vertex always being the middle letter. For instance, ∠ABC signifies the angle formed by the points A, B, and C, with B as the vertex. Essential in various fields of study, such as geometry, trigonometry, and even physics, the angle symbol is a universal mathematical tool contributing to our understanding of the world around us.
Other Names
While the angle symbol (∠) is the most universally recognized notation for angles, it has various names that differ based on context and region. In English, it’s straightforwardly known as the “angle sign” or “angle symbol.” In certain mathematical settings, it can also be called the “angular measure symbol.”
In the context of trigonometry, when denoting a specific angle within a right triangle (opposite/hypotenuse, for example), it’s often referred to as the “Greek letter theta (θ)” or other Greek letters such as “alpha (α)” and “beta (β).” The name may vary, but the concept remains the same, a testament to angles’ ubiquity and essential nature in mathematics.
Angle Symbol Meaning
The angle symbol (∠) is a mathematical symbol used to denote an angle in geometric diagrams. The angle symbol (∠) is primarily used in geometry to denote an angle formed by two rays meeting at a common endpoint or vertex. The symbol resembles a small arc with a vertex point, visually representing the corner or point where two lines meet at an angle.
The angle symbol (∠) is a fundamental component of mathematical notation representing an angle. An angle, in its geometric sense, is a figure formed by two rays sharing the same endpoint, which is called the vertex of the angle. The symbol ∠ visually mirrors this geometric concept, appearing as two lines emerging from a common point.
When used in mathematical expressions, the angle symbol is typically followed by three letters denoting points in the space that form the angle, with the vertex as the middle letter. For example, ∠ABC would signify the angle formed by points A, B, and C, where B is the vertex.
The angle symbol doesn’t denote the measure of the angle on its own; the measurement, usually in degrees or radians, is typically provided in a mathematical problem or derived through calculations.
As such, the angle symbol is crucial in various branches of mathematics and science, including geometry, trigonometry, physics, and engineering. It allows for the accurate expression and understanding of fundamental geometric relationships.
Symbolism of the Angle Symbol (∠)
The angle symbol (∠) carries significant mathematical and broader cultural symbolism. It is not merely a notational convenience but also a symbol loaded with meanings related to perspective, measurement, and understanding.
Representation of Measurement and Precision
In geometry, the angle symbol (∠) represents the precise measurement of the space between two intersecting lines or planes. This measurement is fundamental to understanding the relationships between forms and can symbolize the concept of perspective — how we see and interpret the angles of the world around us. In engineering and architecture, the symbol emphasizes the importance of precision and meticulous planning.
Philosophical and Abstract Symbolism
Philosophically, angles can symbolize divergence or convergence of ideas depending on their orientation. Acute angles might suggest sharpness or quick change, while obtuse angles could represent openness or slow transformation. The angle symbol (∠) encapsulates these ideas, serving as a reminder of how different viewpoints can lead to varied interpretations and conclusions.
Cultural and Educational Implications
Educationally, the angle symbol (∠) is a gateway to deeper mathematical literacy and critical thinking. It encourages learners to explore geometry’s properties and apply them to solve problems, promoting a logical and analytical approach to challenges. Culturally, the symbol signifies knowledge, learning, and the pursuit of understanding, embodying the intellectual endeavor to make sense of our environment.
The angle symbol (∠) is rich with symbolism, extending beyond its practical use in mathematics to represent broader themes of perspective, precision, and philosophical inquiry. Its presence in educational contexts underlines these themes, reinforcing its role as a foundational element in developing analytical and critical thinking skills.
Angle Symbol Unicode
Unicode is a universal character encoding standard that assigns a unique number, or “code point,” to each character used in written languages, enabling their use and recognition across different platforms and programs. From common alphabet letters and punctuation marks to more specialized symbols like those used in mathematics, Unicode ensures consistent representation of text in digital mediums.
The angle symbol (∠) is part of this extensive system. Its Unicode number is U+2220, corresponding to the hexadecimal number 2220 in the Unicode standard.
So, when you’re trying to use this symbol in a digital document, knowing its Unicode can help you insert it correctly, even if your keyboard doesn’t have a direct key.
Angle Symbol Uses
The angle symbol (∠) is extensively used in geometry, trigonometry, and various branches of mathematics and physics where measuring and denoting angles are required. Here’s a detailed exploration of its practical applications:
1. Geometry and Trigonometry
- Geometric Diagrams: The angle symbol is fundamental in geometric diagrams to denote specific angles. This helps clarify instructions and solutions in textbooks, research papers, and educational materials.
- Trigonometric Calculations: In trigonometry, the angle symbol specifies angles within trigonometric functions like sine, cosine, and tangent, which are essential for solving problems involving triangles and periodic phenomena.
2. Educational Tools
- Teaching Resources: Educators use the angle symbol to teach students about the properties and relationships between angles. It is a critical component in illustrating problems and examples in educational materials.
- Exams and Tests: The angle symbol appears frequently in standardized tests and classroom exams to specify the measurement of angles in math problems, ensuring clarity and precision in the questions.
3. Engineering and Architecture
- Blueprints and Design: In engineering and architecture, the angle symbol is used in technical drawings and blueprints to denote specific angular measurements, which are crucial for constructing buildings, machinery, and other structures.
- Simulation and Modeling: Software used in engineering and architectural design often utilizes the angle symbol to input and manipulate angles in models and simulations, ensuring designs are accurate and viable.
4. Computer Graphics and Gaming
- Game Development: In computer graphics and game development, angles are crucial for defining the orientation and rotation of objects and camera views. The angle symbol is used in programming environments to communicate these measurements clearly.
- Animation and Modeling: Animators use angles to position characters and objects precisely, making the angle symbol important in scripting and animation software.
5. Astronomy and Navigation
- Celestial Measurements: Astronomers use the angle symbol to denote angles between celestial bodies or horizon points, essential for tracking movements and positions in the sky.
- Navigation: In navigation, angles plot courses and navigate by celestial bodies. The angle symbol helps in denoting these measurements in navigational calculations and maps.
6. Robotics and Automation
- Movement and Orientation: In robotics, angles are critical for programming the movement of joints and components. The angle symbol is used in programming languages and interface designs to denote these measurements, facilitating precise control over robotic movements.
In conclusion, the angle symbol (∠) is more than just a mathematical notation; it’s a versatile tool used across various fields to communicate and work effectively with angular measurements. Its usage facilitates precision, clarity, and efficiency in many professional and academic settings.
Angle Symbol Examples
The angle symbol (∠) is fundamental in various mathematical and practical applications. To illustrate how it’s used, here are some concrete examples from different contexts:
1. Mathematics Education
- Problem Statement: Consider a triangle ABC where ∠ABC must be calculated based on the lengths of the sides. In this instance, the angle symbol helps specify exactly which angle in the triangle is being discussed.
- Solution Explanation: You might use the law of cosines or other trigonometric relationships to solve for ABC. Using the angle symbol in the explanation ensures clarity when referencing specific triangle angles.
2. Engineering Drawings
- Blueprints: An engineer designing a part may annotate an angle between two intersecting pieces with, for example, ∠154°, indicating the exact angle needed for manufacturing or assembly purposes.
- Construction Plans: In civil engineering, the angle between two roads might be denoted as ∠120° on a plan, guiding construction teams in executing road intersections.
3. Computer Programming
- Graphics Programming: When rotating a sprite in a game, the programmer might specify the rotation by using an angle, such as
rotate(∠45°), to turn the sprite 45 degrees clockwise. - Robotics Control: In a script controlling a robot arm, the angle symbol might be used like
setJointAngle(joint1, ∠90°)to make the joint move to a 90-degree position.
4. Astronomy
- Observation Data: Astronomers might record that two stars are visible in the night sky with an angular separation of ∠15°, indicating how far apart they appear to be from Earth.
- Telescope Alignment: Instructions for aligning a telescope might include tilting the scope ∠45° from the horizon to observe a specific celestial event.
5. Navigation
- Maritime Navigation: A ship’s captain might use the angle symbol in plotting a course, noting that the ship must turn to a bearing of ∠NE 45°.
- Aircraft Piloting: When landing, pilots adjust their approach angle, which might be communicated as adjusting to ∠3° for a gradual descent.
6. Art and Design
- 3D Modeling: Artists working with 3D models might use angles to describe the rotation of parts of the model, such as adjusting a character’s head to look upwards by ∠15°.
- Architecture: When designing a building with a sloped roof, an architect might specify that the roof should tilt at an angle of ∠30° relative to the horizontal plane to ensure proper rain runoff.
These examples highlight the versatility of the angle symbol (∠) across various fields, demonstrating its crucial role in conveying precise angular measurements and facilitating effective communication in mathematical, scientific, and practical applications.
Why is the Angle Symbol Important?
The Angle symbol (∠) holds significant importance due to its fundamental role in numerous scientific and mathematical disciplines. This simple symbol represents a concept that helps us understand and measure the world around us, from the straight lines and sharp corners of architectural structures to the complex movements in celestial bodies.
It’s essential in geometry and trigonometry, providing the foundation for further exploration into shapes, patterns, and relationships between different entities. In physics, it aids in studying forces, movement, and the behavior of light. Communication and comprehension of these critical concepts would be more challenging without the angle symbol.
History of the Angle Symbol
The angle symbol (∠), a concise and visually descriptive notation, has an intriguing history rooted in the development of mathematical notation over centuries. Its journey reflects broader changes in how mathematicians and scholars have sought to communicate complex geometric concepts.
Early Geometric Notation
In ancient civilizations like Egypt and Babylonia, geometry was mainly practical and used for land measurement and architecture. The Greeks, particularly Euclid in his “Elements” (circa 300 BCE), further developed geometric theory, but still without a consistent symbolic notation. Angles were described in words, not symbols, a less efficient method for conveying complex ideas.
Medieval and Renaissance Developments
During the medieval period, Arabic mathematicians made significant advances in geometry, but like their Greek predecessors, they primarily used text to describe angles. The Renaissance renewed interest in geometry, and European mathematicians began to seek more efficient ways to express geometrical relationships, including angles.
The Introduction of Modern Notation
The symbol for an angle (∠) we recognize today did not appear until much later. Mathematical notation began to evolve rapidly during the 16th and 17th centuries, thanks to figures like René Descartes and François Viète, who introduced the systematic use of algebraic symbols. However, the specific notation for an angle took longer to standardize.
The 18th and 19th Centuries
The angle symbol, as it is currently known, was popularized in the 18th century. Mathematicians sought to create a universal language for mathematical concepts to facilitate easier communication and teaching across national and language barriers. The symbol (∠) began to appear in mathematical texts to denote angles, simplifying both the writing and understanding of geometric propositions.
Standardization in the 20th Century
With the advent of modern mathematics and its formalization in education systems worldwide, the angle symbol (∠) became standardized. It was included in the set of mathematical symbols, which were universally taught in schools and used in texts, making them a fundamental element of geometry education.
Today, the angle symbol (∠) is universally recognized in mathematics education, technical fields, and anywhere else geometry plays a role. Its development from descriptive text to a concise symbol illustrates the evolution of mathematical notation from a cumbersome system to one geared toward efficiency and clarity.
This symbol not only helps in the accurate depiction of geometric figures but also facilitates global communication in the scientific community. Its history is a testament to the ongoing quest for clarity and precision in mathematical language.
Origin of the Angle Symbol
The origin of the angle symbol (∠) is a fascinating intersection of typographic innovation and the evolution of mathematical notation. While the concept of an angle has been a fundamental part of geometry since ancient times, the specific symbol we use today to represent an angle has a relatively more modern history.
Early Geometric Concepts
Angles, as a concept, were studied extensively in ancient civilizations, including by the Greeks and Babylonians. These early mathematicians used geometric principles primarily for construction, astronomy, and land measurement. However, their representations of angles were typically described in words rather than symbols, limiting communication speed and clarity in mathematical texts.
Development of Mathematical Notation
It wasn’t until the Renaissance that a more systematic approach to mathematical notation emerged. Mathematicians like Johannes Kepler and others in the 16th and 17th centuries started to develop symbols for mathematical concepts to aid in the clarity and brevity of their work. However, the specific symbol for an angle took longer to appear.
Emergence of the Angle Symbol
The modern angle symbol (∠) was used in the 18th century. Its design is straightforward yet effective: a simple arc with a vertex at its endpoint suggests an angle without using words. The symbol likely evolved from the need to clearly and quickly denote angles in geometric figures, especially in instructional and theoretical texts.
Symbol Standardization
The adoption of the angle symbol (∠) became more widespread with the increased printing of mathematical and scientific textbooks, which standardized many aspects of mathematical notation across Europe. By the 19th century, the symbol was commonly accepted in mathematical texts and was taught as part of the standard school curriculum.
Modern Usage
Today, the angle symbol is ubiquitous in mathematical and engineering diagrams worldwide. Its simplicity and effectiveness in denoting angles have made it a critical component of modern mathematical notation.
The angle symbol (∠) is a product of the gradual evolution of mathematical notation, reflecting a broader trend towards the standardization and simplification of symbols in technical and scientific communication. Its origin illustrates the transition from verbal descriptions to a universal symbolic language in mathematics, facilitating clearer and more efficient communication of complex ideas.
Evolution of the Angle Symbol
The angle sign (∠) as we know it today has been relatively stable in design and application over the past century. Its visual representation—two lines originating from a common point—is intuitively linked to the concept of an angle, likely contributing to the stability of its design. However, the broader concept of representing angles in mathematical notation has indeed evolved. Ancient Greek mathematicians, for example, used letters from the Greek alphabet to represent angles in their work.
As mathematical notation began to standardize globally in the late 19th and early 20th centuries, the current ∠ symbol became widely accepted. This standardization allowed for more coherent communication and comprehension of mathematical ideas across different languages and cultures. While the specific symbol hasn’t changed much recently, its acceptance and usage have grown, underscoring its vital role in mathematics and related fields.
Angle Symbol In Everyday Life
Reflecting on the Angle symbol (∠) reminds us of the power of simplicity and precision. It’s a simple geometric shape, yet it forms the basis of complex mathematical and scientific theories.
This principle can be applied to our everyday lives by remembering that, sometimes, simplicity is key. Whether we communicate, plan our day, or even arrange our workspace, striving for simplicity can often lead to greater clarity and effectiveness.
Last Thoughts
From deep mathematical explorations to its influences in pop culture, the Angle symbol (∠) truly exhibits versatility and ubiquity. It’s not just a notation; it’s a cornerstone of our understanding of the world, embedded in the fundamental fabric of numerous disciplines. We hope this exploration has illuminated the diverse dimensions of this fascinating symbol for you.
Before You Go
Now that you’ve uncovered the fascinating world of the Angle symbol, we invite you to continue exploring the rich universe of symbols—each one a door to new understanding and discovery!
If you’ve enjoyed uncovering the origins and uses of the angle symbol (∠) with us, why not spread the word? Share this post with friends, classmates, or colleagues who might find this blend of mathematical history and practical application as intriguing as yours.
Whether through social media, email, or a casual conversation, discussing what you’ve learned can spark curiosity and further exploration, enriching your shared knowledge and appreciation for the fascinating world of mathematics.
Let’s keep the conversation going and inspire more discoveries!













